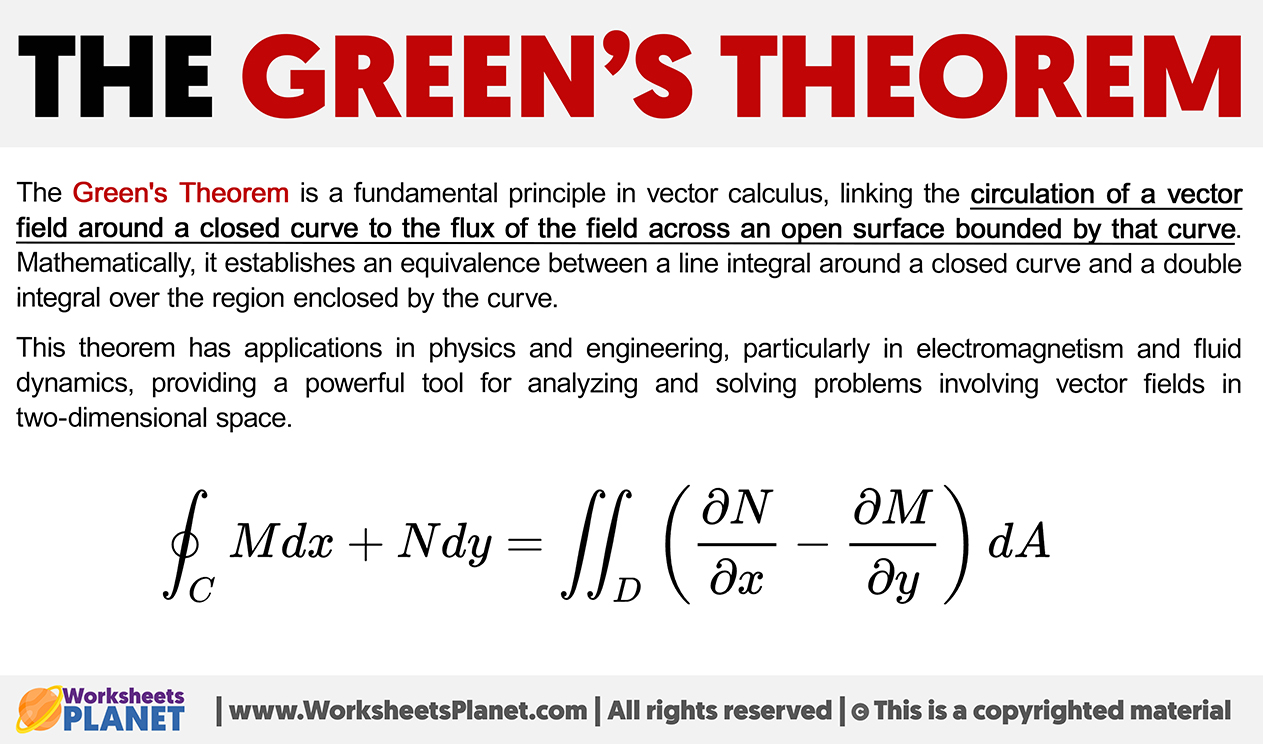
The Green’s Theorem is a fundamental principle in vector calculus, linking the circulation of a vector field around a closed curve to the flux of the field across an open surface bounded by that curve.
Mathematically, it establishes an equivalence between a line integral around a closed curve and a double integral over the region enclosed by the curve.
This theorem has applications in physics and engineering, particularly in electromagnetism and fluid dynamics, providing a powerful tool for analyzing and solving problems involving vector fields in two-dimensional space.