In Math, the division between prime and composite numbers is a fundamental factor since understanding what each one represents, and their usefulness will allow the development of the Fundamental Theorem of Arithmetic.
This theorem, also known as the unique factorization theorem, states that every positive integer greater than 1 is either a prime number or a unique product of prime numbers.
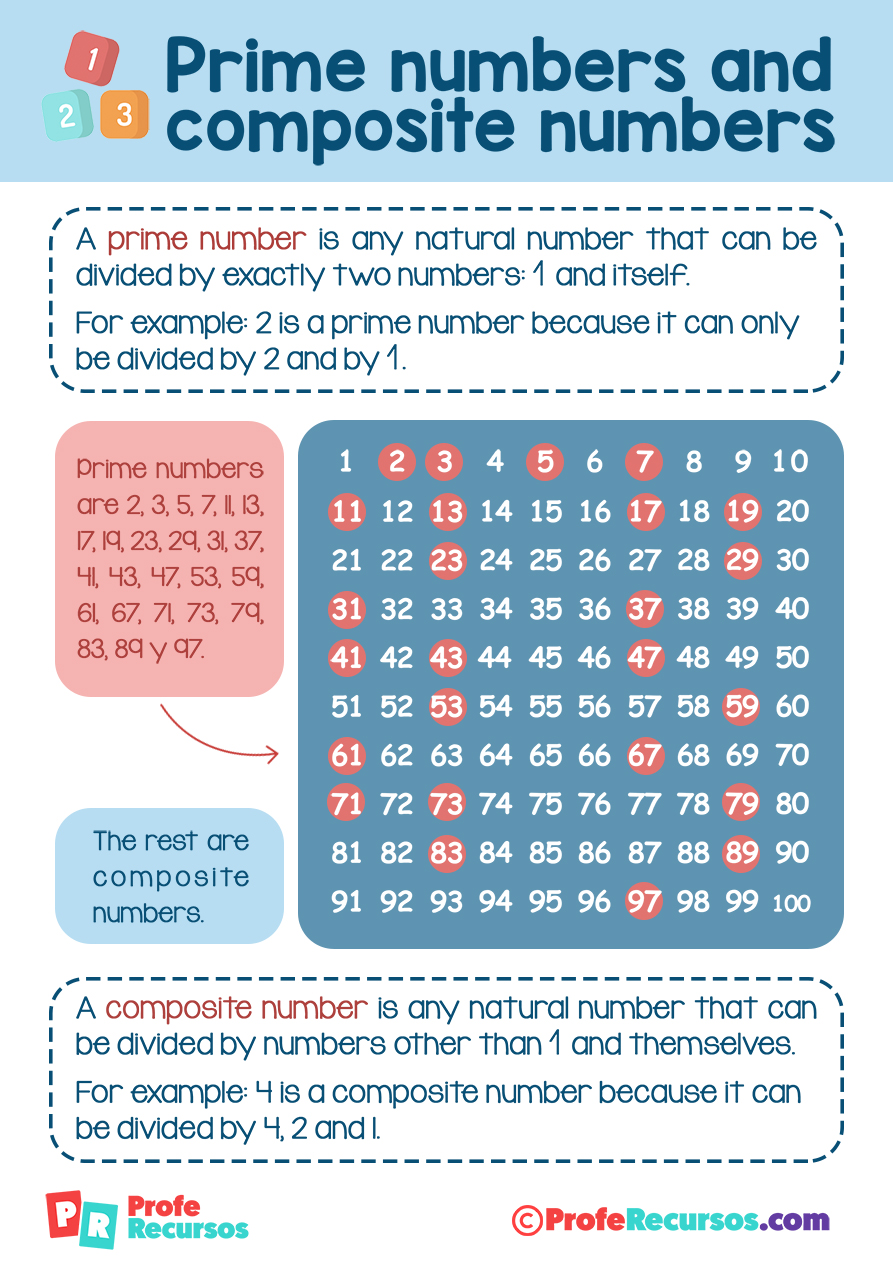
Difference Between Prime and Composite Numbers
Prime numbers are those that are only divisible by themselves, and 1. For example, 7 is a prime number because it can only be divided by 7 and 1.
Another example, 13 is a prime number. As in the previous case, it can only be divided between 1 and 13.
On the contrary, composite numbers are those natural numbers that have some divisor apart from themselves and 1, and, therefore, can be factored. The number 1, by convention, is considered neither prime nor composite.
25 is a composite number; it is divisible by 1, 25, and 5. 14 is also divisible because it can be divided by 1, 2, 7, and 14.

