An angle is a portion of a plane bounded by two rays originating at the same point. The rays are called sides. Their common origin is the angle’s vertex. We can represent angles with a lowercase letter, like a or b, or sometimes with a Greek letter like (α).
Another definition: An angle is the part of the plane between two rays that have a common origin. The rays are called sides, and the common origin is called the vertex. To represent an angle, we draw an arc between the rays and place a lowercase letter or a Greek letter. It is written «∡ α».
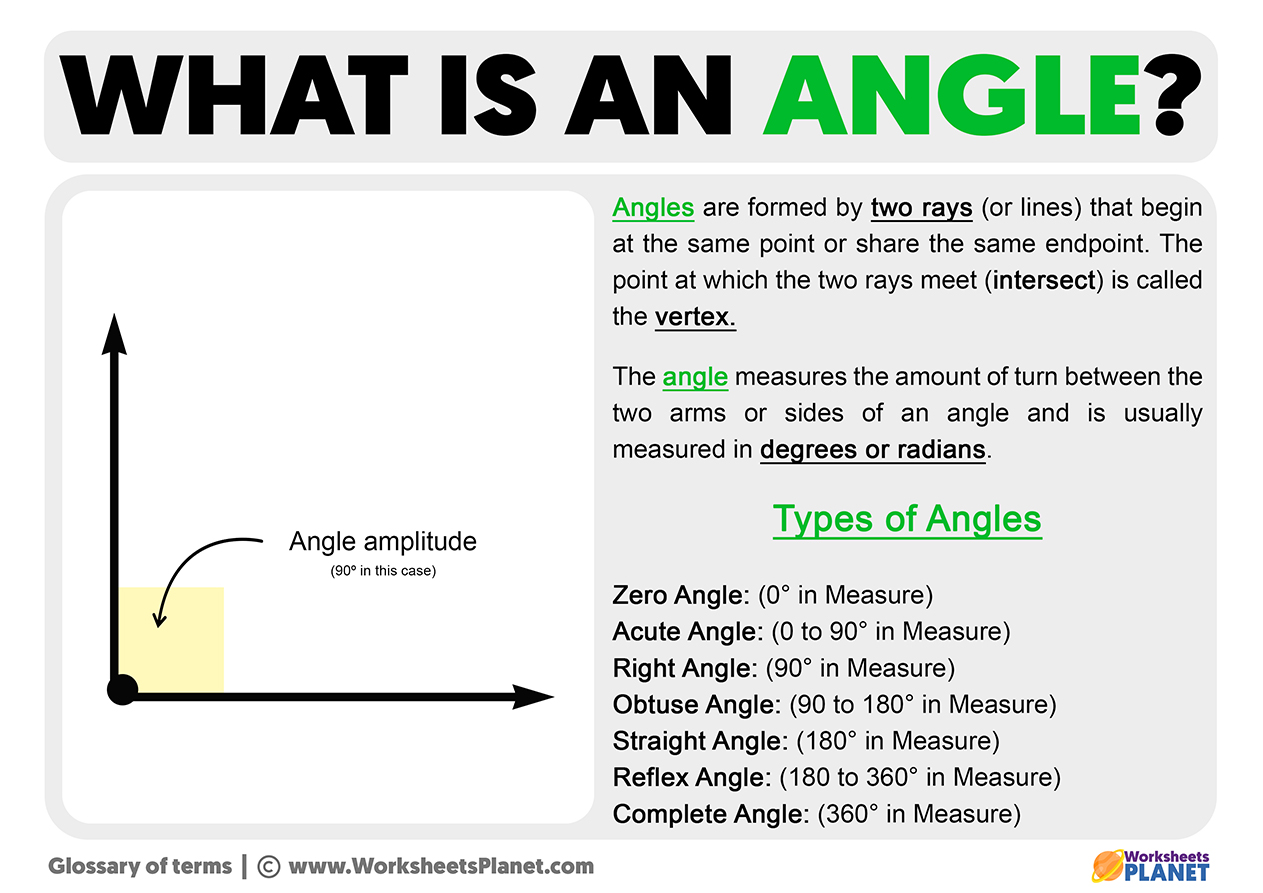
Types of Angles
- Null angle: is an angle of 0°; the rays coincide.
- Straight angle: it is an angle of 180°.
- Right Angle: It is an angle of 90°.
- Acute Angle: An angle that measures less than 90°.
- Obtuse Angle: An angle that measures more than 90°.
- Complementary Angles: Two angles are complementary when they add up to 90°.
- Supplementary Angles: Two angles are supplementary when they add up to 180°.
- Opposite angles by the vertex: Are those with the same vertex, and the sides of one are the rays opposite the other. These are congruent. That is, they have the same measure.
- Consecutive Angles: Two or more angles are consecutive when they have the same vertex and a side in common but do not overlap.
- Adjacent Angles: Two angles are adjacent when they are consecutive, and their non-common sides form a straight angle. These types of angles are supplementary because:
Measurement of an Angle
The amplitude of an angle is measured with a protractor graduated in degrees. The number marked is called the measure or amplitude of the angle. Two angles are congruent when they have the same measure. Three types of measurements are used to measure the amplitude of an angle:
- Sexagesimal degrees.
- Radians
- Grad degrees.

