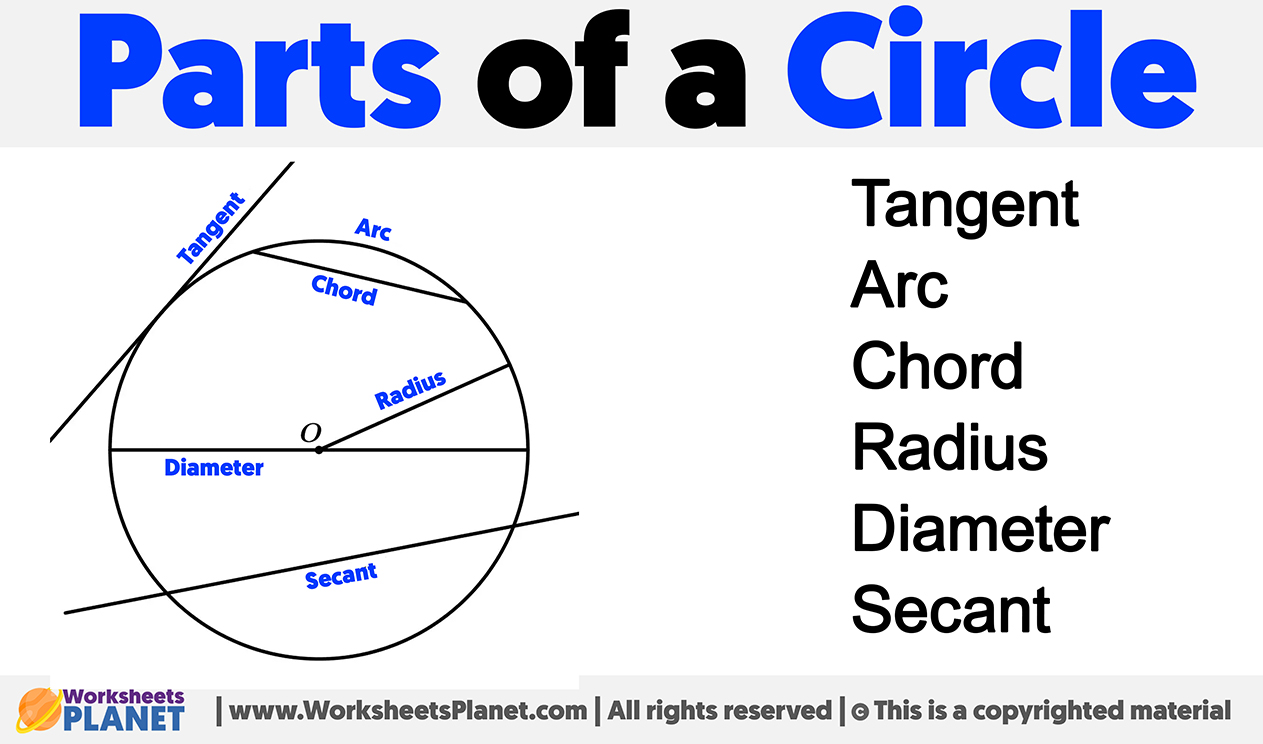
A circumference is a closed curve that represents the boundary of a circle. Understanding its components is crucial for geometry and various mathematical applications. Here’s a brief explanation of each part:
Tangent: A tangent is a line that touches the circumference at exactly one point without crossing it. This point of contact is perpendicular to the radius at that point. Tangents play a key role in trigonometry and calculus, aiding in the analysis of circular motion and curves.
Arc: An arc is a curved segment of the circumference, delineated by two distinct points on the circle. It is measured in degrees and is a fundamental concept in geometry. Arcs can range from minor arcs, which are less than 180 degrees, to major arcs, which are greater than 180 degrees.
Chord: A chord is a straight line segment connecting two points on the circumference. The diameter is a special type of chord that passes through the center of the circle. Chords are crucial in geometry, and their properties are extensively used to analyze circles and solve mathematical problems.
Radius: The radius is a line segment that connects the center of the circle to any point on its circumference. All radii in a given circle are of equal length. The radius is fundamental for calculating the area and circumference of a circle and is a pivotal parameter in various mathematical formulas.
Diameter: The diameter is the longest chord in a circle, passing through the center and connecting two points on the circumference. It is precisely twice the length of the radius. The diameter is a fundamental measurement in geometry, often used to calculate other circle properties.
Secant: A secant is a straight line that intersects the circumference at two distinct points. It is broader than a chord, as it extends beyond the circle. The term is often used in trigonometry and geometry to analyze the relationships between angles formed by secants and other circle elements.